 |
Znanstveno-stručni
časopis Hrvatskog društva za geometriju i grafiku Scientific and Professional Journal |
|
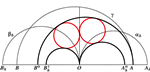
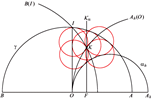
| N. Le, N. J. WildBerger (n.h.le@unsw.edu.au, n.wildberger@unsw.edu.au)
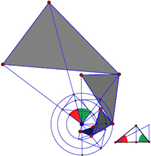
Incenter Circles, Chromogeometry, and the
|
 Article in PDF.
Article in PDF.
| Hiroshi Okumura (hiroshiokmr@gmail.com)
The Arbelos with OverhangWe consider a generalized arbelos consisting of three semicircles with collinear centers, in which only two of the three |
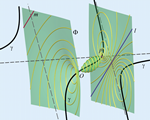
| Boris Odehnal (boris.odehnal@uni-ak.ac.at)
Distances and Central ProjectionsGiven a point P in Euclidean space R3 we look for all points Q such that the length PQ of the line segments PQ from P to Q equals the length of the central image of the segment. It turns out that for any fixed point P the set of all points Q is a quartic surface \Phi. The quartic \Phi carries a one-parameter family of circles, has two conical nodes, and intersects the image plane p along a proper line and the three-fold ideal line p2 of \pi if we perform the projective closure of the Euclidean three-space. In the following we shall describe and analyze the surface \Phi.
|
|
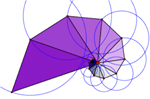
| Gunter Weiss, Sybille Mick (weissgunter@hotmail.com, mick@tugraz.at)
Non-standard Visualizations of Fibonacci Numbers and the Golden MeanFibonacci numbers and the Golden Mean are numbers and
thus 0-dimensional objects. Usually, they are visualized in
the Euclidean plane using squares and rectangles in a spiral arrangement. The Golden Mean, as a ratio, is an affine
geometric concept and therefore Euclidean visualizations
are not mandatory. There are attempts to visualize the
Fibonacci number sequence and Golden Spirals in higher
dimensions [11], in Minkowski planes [12], [4] and in hyperbolic planes (again [4]). The latter has to replace the
not existing squares by sequences of touching circles. This
article aims at visualizations in all Cayley-Klein planes and
makes use of three different visualization ideas: nested
sets of squares, sets of touching circles and sets of triangles that are related to Euclidean right angled triangles. |
|
| Andrej Novak, Andrija Štajduhar (andrej.novak@unidu.hr, astajd@astajd.com)
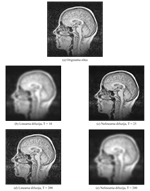
Application of Linear and Nonlinear Heat Equ-
|
|
| Andrea K. Myers-Beaghton, Alan L. Myers (a.beaghton@imperial.ac.uk, amyers@seas.upenn.edu)
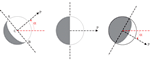
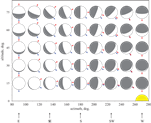
The Moon Tilt IllusionThe moon tilt illusion is the startling discrepancy between the direction of the light beam illuminating the moon and the direction of the sun. The illusion arises because the observer erroneously expects a light ray between sun and moon to appear as a line of constant slope according to the positions of the sun and the moon in the sky. This expectation does not correspond to the reality that observation by direct vision or a camera is according to perspective projection, for which the observed slope of a straight line in three-dimensional space changes according to the direction of observation. Comparing the observed and expected directions of incoming light at the moon, we derive a quantitative expression for the magnitude of the moon tilt illusion that can be applied to all configurations of sun and moon in the sky.
|